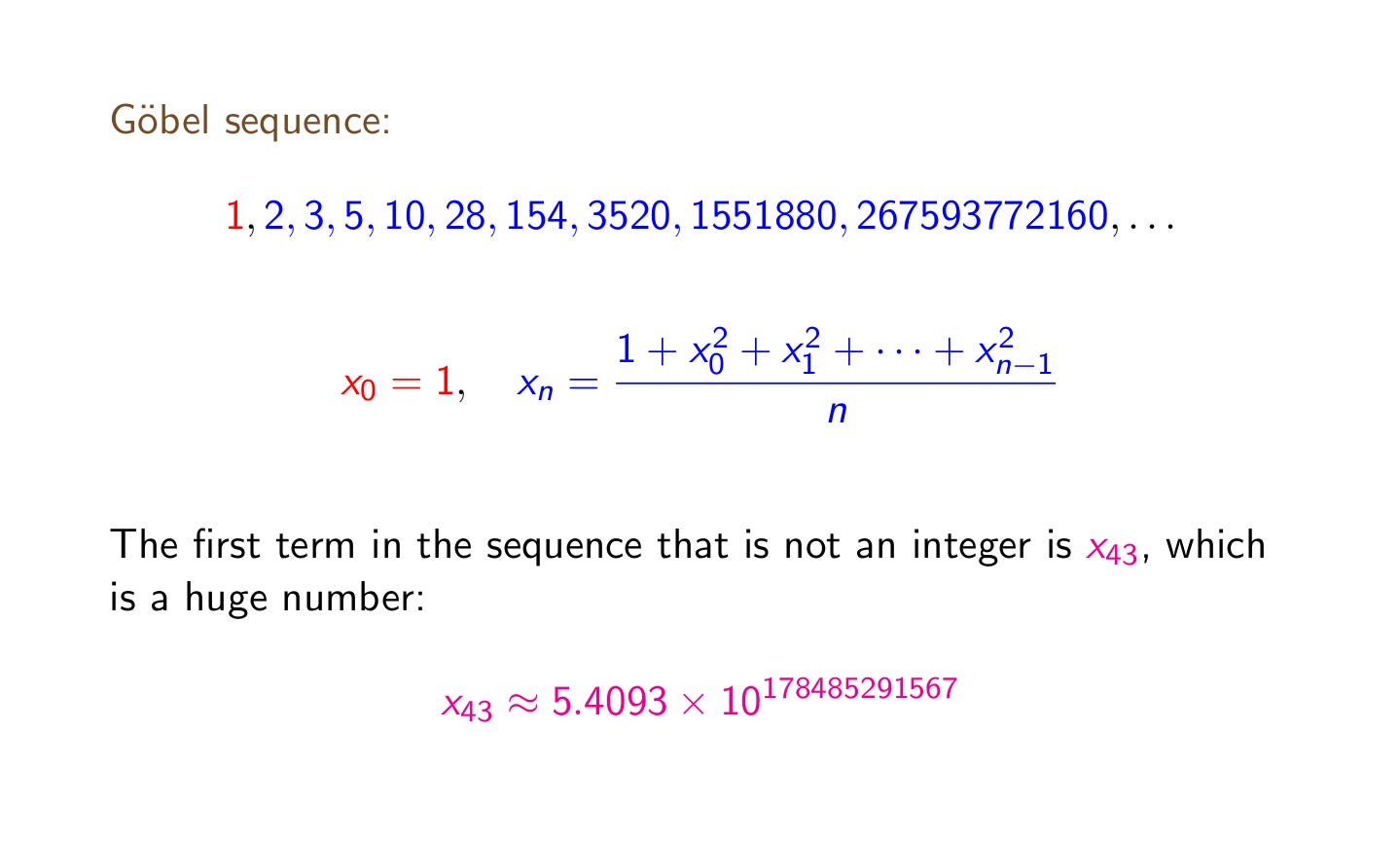
The Göbel sequence (x_0, x_1, x_2, …) is an infinite sequence of numbers given by the formula shown above. Although there is no reason for these numbers to be whole numbers, it turns out that the first 43 of them are, but the 44th term, x_43, is not. Checking this is not as easy as it sounds, because x_43 is larger than the number 5 followed by 178,485,291,567 zeros!
The Göbel sequence is named after Fritz Göbel, who introduced it in the 1970s. Calculating the first few terms of the Göbel sequence is not hard. The first term, x_0, is 1. The (n+1)-st term of the sequence, x_n, is obtained by squaring each of the first n terms, adding them up, adding 1 and finally dividing by n. The case n=5 is shown in the picture above. There is no reason that the last step of dividing by n should result in an integer answer, but for some reason it often does.
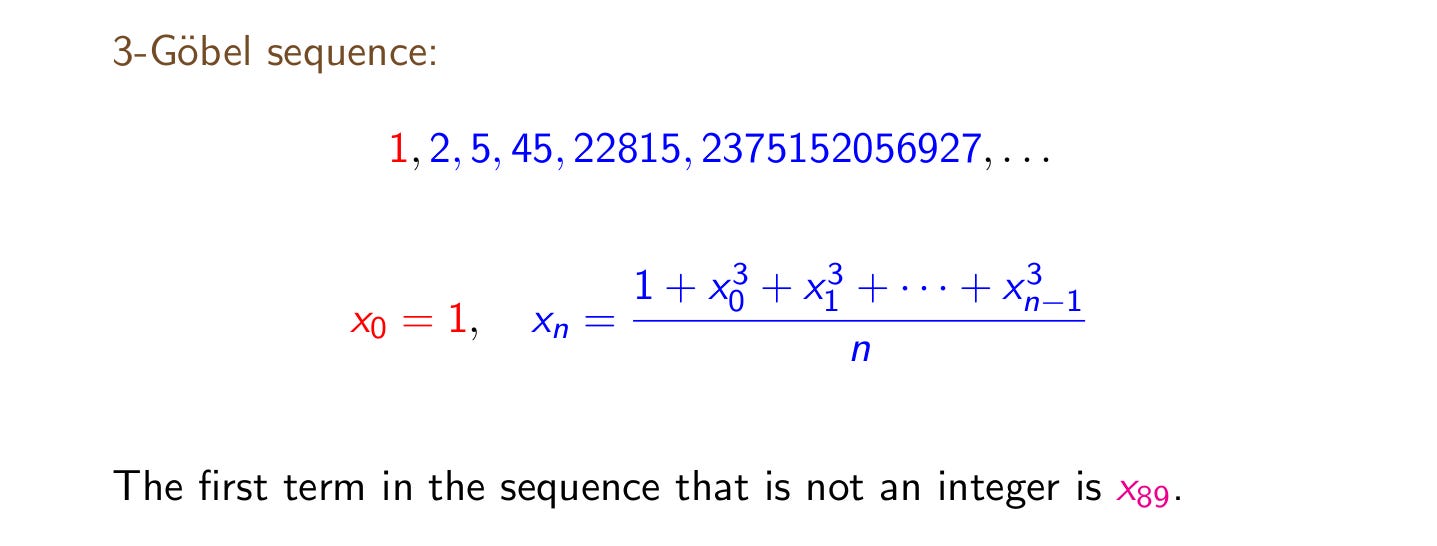
The 3-Göbel sequence is a version of the Göbel sequence where the squares of previous terms are replaced by cubes (third powers). The first few terms are 1, 2, 5, 45, 22815, and 2375152056927. This sequence grows even more quickly than the original sequence, and contains even more integers. It turns out that the first 89 terms of the 3-Göbel sequence are integers, but the 90th term is not.
More generally, for any integer k ≥ 2, the k-Göbel sequence is given by the formula above, replacing the squares in the original Göbel sequence by k-th powers.
Remarkably, the k-Göbel sequences all begin with many integer terms. As we mentioned above, the original 2-Göbel sequence starts with 43 integer terms, and the 3-Göbel sequence starts with 89 integer terms. What happens in general is shown in the table above, which shows that N_6 = 19 and N_31 = 1077. This means that the 6-Göbel sequence starts with 19 integer terms before producing a non-integer, and the 31-Göbel sequence starts with a whopping 1077 integer terms before producing a non-integer. The numbers N_k in the table seem to exhibit some structure: for example many (but by no means all) of the N_k are prime numbers, such as 19, 43, 89, and 1559.
Seisū-tan (“the tale of integers”) is a Japanese manga by Doom Kobayashi and Shin-ichiro Seki. A frame from the manga, shown above with a Google translation, recently raised the question of what the minimum possible value of N_k is. This question is answered in the short new paper How long can k-Göbel sequences remain integers? by Rinnosuke Matsuhira, Toshiki Matsusaka, and Koki Tsuchida. The answer is that the minimum possible value of N_k is 19, which means that, no matter how big k is, we can guarantee that the k-Göbel sequence begins with at least 19 integer terms. In some cases, such as that of the 6-Göbel sequence, this minimum value of 19 integer terms is achieved. The paper also proves that there is no value of k for which N_k = 41.
Another question to ask is: are the values of N_k always finite? To ask this another way, is there a value of k for which the k-Göbel sequence consists entirely of integers? Even if the values of N_k are always finite, is there a bound to how big the values can get? The authors of the paper mention that both these questions are posed in the manga, but they remain open problems.
Picture credits and relevant links
The table of values of N_k comes from the paper by Matsuhira, Matsusaka, and Tsuchida.
The excerpt from the manga, which also appears in the paper, comes from Episode 3 of Seisū-tan. The translation (from Japanese to U.S. English) is by Google Translate.
The other graphics are my own work.
Wolfram MathWorld has an article by Eric W. Weisstein on Göbel’s Sequence.
The On-Line Encyclopedia of Integer Sequences has entries on the Göbel sequence, the 3-Göbel sequence, and the table of N_k for various k.