Rubik’s Cube is a well-known combination puzzle that was invented by Ernő Rubik in 1974. What makes the cube interesting, both as a puzzle and as a mathematical object, is the enormous amount of symmetry it has. The puzzle can be permuted in approximately 43 quintillion (4.3×1019) ways, and a stack of this many cubes would form a tower 261 light years high.
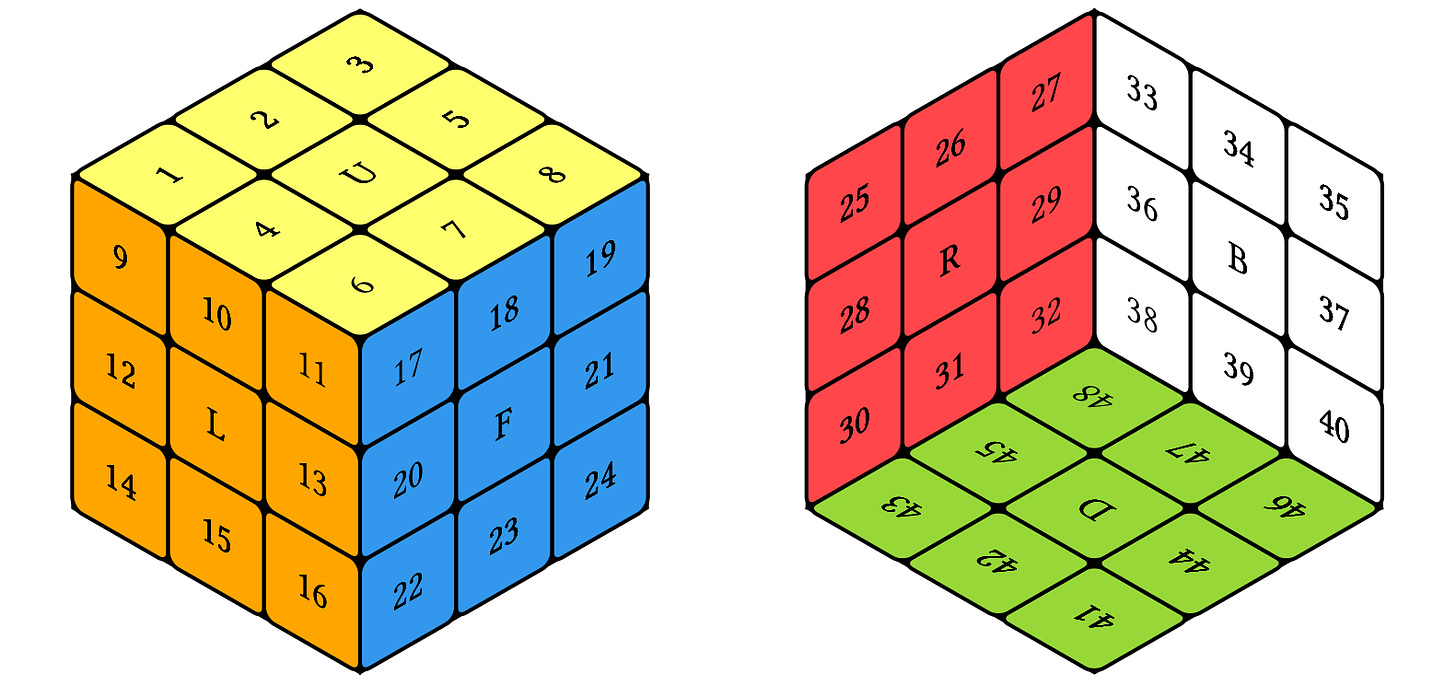
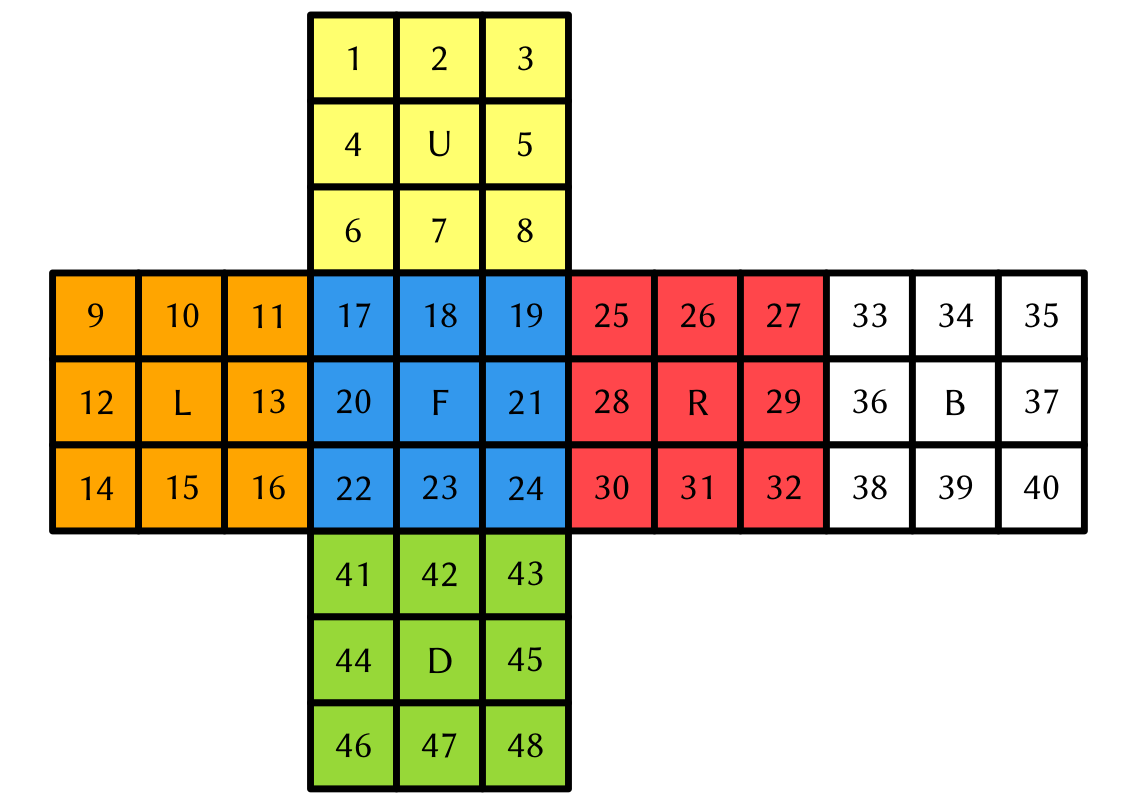
Rubik’s Cube has six faces, coloured red, orange, yellow, green, blue, and white. The cube has 26 pieces: 8 corner pieces, 12 edge pieces, and 6 centre pieces. In the diagram above, the faces are labelled L, R, U, D, B, and F, which stand for left, right, up, down, back, and front, respectively. We consider the cube remaining in a fixed orientation, so that for example the blue centre piece, which is labelled F, continues to face towards the front. One sees from the net of the cube, shown below, that the three opposite pairs of faces are red/orange, yellow/green, and blue/white.
We define the transformations T1,T2,T3,T4,T5, andT6 to be the rotations by 90° clockwise of the yellow, orange, blue, red, white, and green faces, respectively. These can be regarded as basic transformations in the sense that every other transformation is a combination of them. For example, an anticlockwise rotation by 90° can be accomplished either by applying (T1)3, meaning applying T1 three times in succession, or by applying the inverse of T1. Similarly, the transformation (T5)2 has the effect of rotating the white face by 180°.
Mathematicians express this by saying that the set {T1,T2,T3,T4,T5,T6} is a set of generators for the group of symmetries of Rubik’s cube. In fact, the group of symmetries can be generated by a smaller set {α, β}, given by the formulae shown above.
The exact formula for the number of symmetries is shown above. To understand where this comes from, it helps to consider the effect of each symmetry on the 8 corner pieces and the 12 edge pieces, as shown below. An important feature of these pieces is that each each corner piece has three possible orientations, and each edge piece has two possible orientations. It turns out that the 8 corner pieces can be rearranged into any order using a sequence of legal moves. Furthermore, it is possible to orient any 7 of the 8 corner pieces arbitrarily, but the orientation of the 8th corner is then fixed. This gives a total of 8! × 37 combinations (where ! denotes factorial).
There would be 12! ways to rearrange the edge pieces if every possible permutation of edges were achievable, but this turns out not to be the case. Any permutation of n objects can be achieved by repeatedly applying transpositions, meaning repeatedly choosing a pair of objects and swapping them. A permutation is called even (respectively, odd) if it requires an even (respectively, odd) number of transpositions. If n is at least 2, then it turns out that exactly half the permutations of n objects are even and half are odd. In the case of the Rubik’s cube, it turns out that a sequence of moves produces an even permutation of the 12 edge pieces if and only if it produces an even permutation of the 8 corner pieces. This gives a total of 12!/2 permutations of the edges once a permutation of the corners has been fixed. It also turns out that 11 of the 12 edge pieces can be oriented arbitrarily, but then the orientation of the 12th piece is fixed. This gives a further factor of 211 permutations, leading to the formula 8! × 37 × 12!/2 × 211 = 43,252,003,274,489,856,000 for the total number of symmetries.
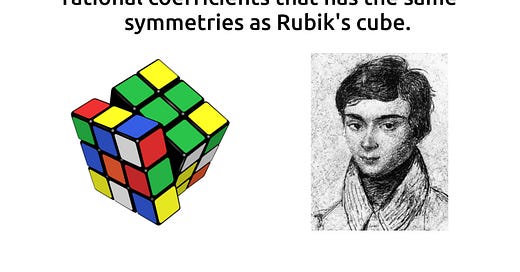
Group theory, which is the mathematical study of symmetry, is also useful in studying the roots of polynomials. Galois theory, which is the study of such symmetries, is named after Évariste Galois (1811–1832). Galois (shown above) died in a duel at the age of 20, but fortunately he had the foresight to write all his ideas down shortly before his death. As a simple example of Galois theory, consider the polynomial equation x2 – 4x +13 = 0, working over the rational numbers, Q. Using the quadratic formula, we find that the roots of this equation are 2 + 3i and 2 – 3i. It is significant that these roots are complex conjugates of each other, meaning that they are of the form a + bi and a – bi. It is possible to make precise the statement that the roots of the polynomial x2 – 4x +13 have two symmetries: the identity map and complex conjugation, and the Galois group has two elements.
A major unsolved problem in algebra is the inverse Galois problem, which asks whether any finite group of symmetries can be realized as the symmetries of a polynomial whose coefficients are rational numbers. The recent paper Rubik's as a Galois' by M. Mereb and L. Vendramin proves that the answer is yes in the case of symmetries of Rubik’s Cube. In other words, there is a polynomial with rational coefficients whose roots have the same symmetries as the Rubik’s Cube. Even better than that, the paper gives an explicit example of such a polynomial, namely the one shown above.
Picture credits and relevant links
The picture of Rubik’s Cube is by Booyabazooka and appears on WIkipedia.
The three diagrams of the Rubik’s Cube come from the paper by M. Mereb and L. Vendramin.
The portrait of Évariste Galois appears on Wikipedia.
The other diagrams are my own work.
Substack management by Buzz & Hum.


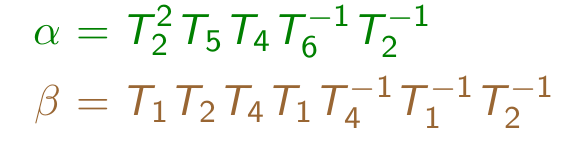
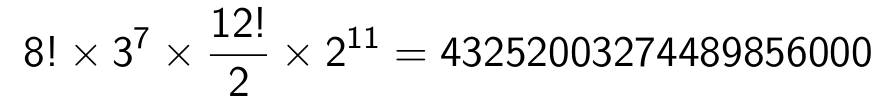



There are Cayley graphs for the Rubik's cube, however is it possible to generate Cayley graphs from Galois groups and rings?
Really appreciated the excellent analysis and discussion of Rubik’s cube and its generalization.